Measure Twice...

We all make mistakes, and with so many projects on the go I probably make more than most. Although it is easy enough to sweep them under the rug and move on that wouldn't be right. This blog isn't just about my successes. Mistakes are educational. I will share two that I recently discovered. 

Consider this an opportunity to learn, and perhaps you will be better prepared to avoid doing the same. With a small dollop of humility let's dive into these latest screw-ups.

Blazing the wrong trail

I mentioned in an article last month that I started clearing a path through the bush for the new east-west reversible Beverage. The west termination is a large tree within the tree line that separates the bush from a hay field. The tree line is also the home for the north-south reversible Beverage. From that tree there is another tree 140 meters due east that was my guide. The full length of the Beverage will be 160 meters.

I selected and surveyed the route with the help of a friend. Guided by a compass we spent a couple of hours picking the best route. Working on my own a few days later, I placed markers every 30 meters along the selected route. I spent several hours work spread over the next couple of weeks, again on my own, cutting down or trimming the bushes and trees that were directly under or too close to the route.

As I mentioned in that article, the path was not perfectly straight. I headed back into the bush to straighten the route. Armed with my compass I carefully sighted to the eastern tree. It wasn't due east. I played with the compass because it was very cold and I suspected that it was sticking. The tree remained stubbornly north of east. Slowly the truth of the matter seeped into my consciousness.

Not too far to the north there is a similar looking tree. I trudged through the snow and bushes and checked again. The tall tree in the distance was now exactly east of my position. I should have marked the tree rather than rely on memory. They all look alike to me. I emailed my friend to blame him for not coming over to check my work. He was not sympathetic.

Most of the desired path is either clear of big trees and, at the eastern end, there is cleared area I can still use. It is no disaster and the bush I cut has no value. It is new growth that naturally sprouted in what had been a tended field decades ago. 

I have more hours of work ahead before I can string the Beverage wire. That work is being delayed by higher priority projects, the pandemic and unseasonably cold weather.

Equivalent diameter of clamps

This one is an old mistake. I discovered it while designing the 10 meter yagis that are on my 2021 project plan. Discovering the mistake was a relief in a way because it explains an anomaly that I discussed on the blog over a year ago. At the time I put it down to a discrepancy between the software model and the physical antenna.

After adjusting the gamma match of the first 5-element 15 meter yagi the SWR curve was very good but not quite in accord with the model. The yagi was set 50 kHz higher than than the design. This was determined by the knee in the impedance curve at the top end of band. This is a good tell in most big yagis for where the antenna is centred because the impedance drops sharply as the frequency of maximum gain is approached.

A quick calculation led me to lengthen every half element by ¼". This small adjustment brought the measured SWR curve into agreement with the model. I thought no more of it at the time since the discrepancy was tiny and no software model perfect mirrors reality.

Recently I built a model for the 10 meter yagis that I plan to build and install later this year. The model deviated from the design in the ARRL Antenna Book. The error was small and at first I was prepared to adjust the element lengths and continue. A closer look revealed a puzzle.

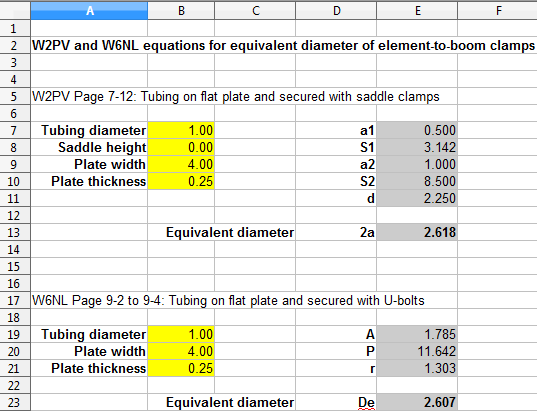
I first modelled a single element using the published dimensions for the element and element-to-boom clamp. While copying the dimensions onto a model of a 15 meter yagi element (to save data entry) I noticed that the effective diameter I used from the 15 meter yagi was different from that in book. That was odd since the clamp design is the same except for the diameter of the centre tube. The 10 meter centre tube diameter in the ARRL Antenna Book is ¾" versus 1" for my 15 meter, yet the effective diameter shown in the book is larger. That isn't possible.

To be exact, my calculation for the 1" tube sitting directly on a 4" × ¼" plate was 1.637". The value in the book is 2.405". I was pretty sure the equations used were the same ones, coming from W6NL's derivation in his Physical Design of Yagi Antennas book (out of print). I wanted to check my calculation but I could find no record of it. It was time to open the book and start from scratch.

The diagram comes from Figure 9-2 in W6NL's book. There are several pages of diagrams and equations about many styles of element-to-boom clamp and the derivation of their respective diameters. Decades ago I used the equations produced by W2PV in his book Yagi Antenna Design (also long out of print). W6NL discusses those results and his, noting that although in most cases the difference is small it can be significant. It was time to sit down to work through the mathematics (again).

For the depicted clamp design the equations are straight-forward, though first appearances may be deceiving if, like many, you have a mathematics allergy. Look at each term in isolation and if you know any geometry at all you'll be able to figure out what's going on.

The area: A = πD²/4 + tW

The perimeter: P = πD + 2(W + t)

The effective radius: a_e = ½[SQRT(A/π) + P/(2π)]

The effective diameter: D_e = 2a_e

If you haven't yet figured it out, here's a hint. The area of the cross-section shown above is the sum of areas of a circle and a rectangle. The same is true of the perimeters. The effective radius is...mmm...sort of half the geometric mean or average of the two. The effective diameter comes from removing the halving operation. Clear as mud? Don't worry about it. It's an approximation that works well in practice, as Dave W6NL assures us.

Riffling through my files I found a spreadsheet with the W2PV equations. I had none for the revised W6NL equations so I added them to the spreadsheet. Having both the old and new equations serves as a sanity check. 

Note that the W2PV equations include saddles on the clamps that raise the tube above the plate. I don't have the equivalent for the W6NL equations. I don't need them, and most don't because it is typical to use heavy wall tube at the centre of the element. A u-bolt is sufficient to hold the tube without risk of crimping it and there is little danger of element slippage.

The value of 1.637" I previously calculated was far too low! I vaguely recall thinking that since the value is intermediate between the tube diameter and plate width it was probably correct. I did not confirm the calculation, not even checking against the W2PV equations spreadsheet.

The error affects the antenna in proportion to the length of the clamp and in inverse proportion to the wavelength. To confirm that my spreadsheet is generating correct results I entered the dimensions for those in the ARRL Antenna Book and I got almost exact agreement with the W6NL equations. 

The funny thing is that Dave Leeson himself pointed me to where I could find a spreadsheet with the equations. I did download it but since it was for an ancient bit of software and had to be converted I decided not to bother and did the calculation myself. I may have done with nothing fancier than a calculator. That was very foolish of me, and especially for not comparing my calculations with the correctly implemented W2PV equations.

With the error identified and corrected I returned to the model of the 5-element 15 meter yagi. Here is the SWR plot of the original design with the 1.637" erroneous equivalent diameter.

Keeping the element tips at their original length I modified the diameter of the 6" long clamps to the new 2.6" value. The SWR was again plotted across the band.

That is just about what I measured in the field and had to correct by lengthening the elements. Next I lengthened the element halves in the model by ¼", just as I did to tune the built antenna.

Lovely. There is no discrepancy between the model and the built antenna; the problem was human (ham) error. The software did exceptionally well to achieve accuracy far better than the misleading ¼" correction. I should learn to trust my software and the experts more than unverified calculations done by hand.

The enduring lesson: measure twice and adjust (or cut) once. As antennas grow larger these avoidable mistakes become more difficult to correct.